|
Mirror Logic and the Algebra of Abstract Groups | |
|
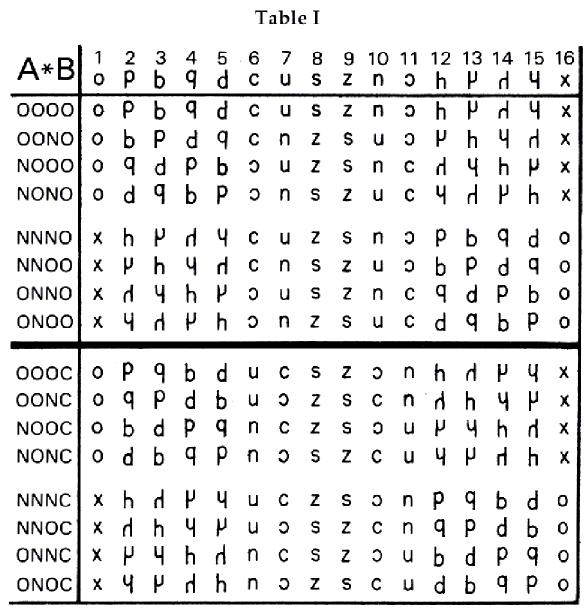
All of this runs head on into the algebra of abstract groups. All of this forces a certain kind of behavior on the logic alphabet. The flip-mate-flip and flip rules will become four mirrors and, under the four rule-governed mirrors, the 16 LSs will be forced to act all alike. This will lead to consequences that are deep and very rich. What follows gives only a small part of the story. Now we have it! Table I is the new multiplication table. Akin to Arabic numerals and the use of zero, the 4-tuple (OOOO) is acting both as the rule-free condition and as a combinatory identity element. The 16 combinations of the four rules along the side (N, N, N, C), which line up with the 16 combinations of the four mirrors, f-m-f and f in that order, are acting on all of the 16 LSs across the top (A * B). This (16 x 16) table is loaded with transformational symmetry. It contains layers of abstract structure. Rarely in a lifetime do we stand in enough light to become aware of the underlying networks of interrelations, always present, usually ignored, and now laid bare. Again, without exception, all and every one of the 16 LSs must obey the same symmetry rules. An easy way to learn about Table I is to notice that Arabic numerals encourage the use of models, such as an abacus and a slide rule. The Romans, in spite of their numerals, did use an abacus; they did not, however, have a slide rule. Modern logic, even more limited than the Romans, does not use hand-held models with which to make logical calculations. In contrast, the logic alphabet is a notation that stumbles all over itself, so many are the models. | |
|
. | |
|
| |